We can solve this question as follows:
• We want to choose 2 colors ,without replacement, from 4 colors.
Then, we have:
1. If the order of the choices is relevant:
We can use the next formula:
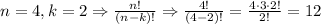
Therefore, we can choose 2 colors, without replacement, from the 4 colors, if the order of choices is relevant in 12 different ways.
2. If the order of the choices is not relevant:
We can use the next formula:
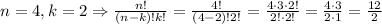
And finally:

Therefore, we can choose 2 colors, without replacement, from the 4 colors, if the order of the choices is not relevant in 6 different ways.