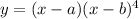ANSWER;
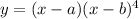
EXPLANATION;
Here, we want to get the equation of the function
From the question, we have that the function is of degree 5. What this mean is that it is expected to have 5 roots which may (with multiplicities) or may not be the same
From the question, we have x = a as a root, with a bounce off at x= b
This mean these two points are the roots
To bounce off simply mean to touch and change direction
Thus, it means to have touched and bounced off b, b is of the bigger multiplicity with the multiplicity of a being one only
Thus, in the factored form, we have the function as;