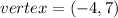We are given the following quadratic equation
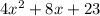
Let us apply the completing the square method to the given equation.
Take the square of the half of the middle term coefficient (that is 8)
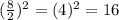
Add and subtract this value from the given equation
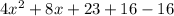
Re-write the equation as below
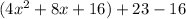
The terms in the parenthesis will be a perfect square so we can factor it out as
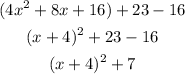
Finally, compare this equation with the vertex form given by
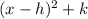
So, we have
h = -4
k = 7
The vertex (h, k) = (-4, 7) is the minimum point of the given quadratic equation