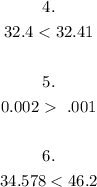Question

Use the greater than > , less than <, and the equal to = symbols to compare each set of decimals

Answer 1

To solve the present problem, we need to remember some properties of the number, such as:

`\begin{gathered} 1.2=1.20=1.200000 \\ .1=0.1 \end{gathered}`

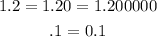
From this, we are able to determine the following:

`\begin{gathered} 32.4=32.40\to32.4<32.41 \\ \text{because .40<.41} \end{gathered}`

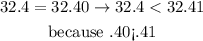
From this, we are able to answer 4. is <

About number 5. we have:

`\begin{gathered} .001=0.001\to0.002>.001 \\ \text{because 2 > 1} \end{gathered}`

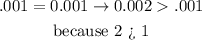
This allows us to answer number 5. as >

About number 6. we can just check the integer part of the numbers because 34<64, and from this, we answer 6. is <

From the above solution, we have the following answers:

`\begin{gathered} 4. \\ 32.4<32.41 \\ \\ 5. \\ 0.002>\text{ }.001 \\ \\ 6. \\ 34.578<46.2 \end{gathered}`