A parallelogram must have equivalent opposite interior angles.
We know that intern angles are represented by the following expression:
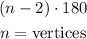
Then, for a parallelogram:
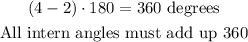
We can create the following equation to find the value of ?:
Let ?=x be the missing angle
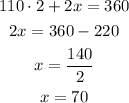
The missing angles have a measure of 70°.