Question

Solve the triangle. (Do not round until the final answer. Round angles to the nearest degree and side lengths to the nearest tenth of a unit.)C=___∠A=___∠B=___

Answer 1

`\begin{gathered} c=7.6 \\ \angle A=37 \end{gathered}`

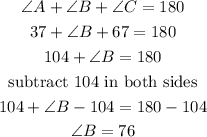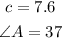
Step-by-step explanation

to solve this we need to use the cosine law

Law of cosines says

`\begin{gathered} a^2=b^2+c^2-2bc\cdot\cos (A) \\ b^2=a^2+c^2-2ac\cdot\cos (B) \\ c^2=a^2+b^2-2ab\cdot\cos (C) \end{gathered}`

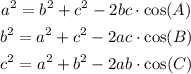
then

Step 1

find c

Let

`\begin{gathered} b=8 \\ a=5 \\ \angle C=67 \end{gathered}`

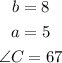
now, let's find c

`\begin{gathered} c^2=a^2+b^2-2bc\cdot\cos (C) \\ \text{replace} \\ c^2=5^2+8^2-2\cdot5\cdot8\cdot\cos (67) \\ c^2=25+64-31.25849028 \\ c^2=57.74 \\ \text{square root in both sides} \\ √(c^2)=√(57.74) \\ c=7.598 \\ \text{rounded} \\ c=7.6 \end{gathered}`

Step 2

now,let's find the angle A

Step 3

Finally,let's find angle B

we can use the formula as we did in step 2 to find angle A, but also we can use this fact:

the sum of the internal angles in a triangle equals 180 ,so

`\angle A+\angle B+\angle C=180`

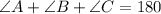
replace and solve for angle C

`\begin{gathered} \angle A+\angle B+\angle C=180 \\ 37+\angle B+67=180 \\ 104+\angle B=180 \\ \text{subtract 104 in both sides} \\ 104+\angle B-104=180-104 \\ \angle B=76 \end{gathered}`