Question

13. Write an equation for a quadratic function whose graph has a vertex at (-1, 3) and goes through the point (2, 4). Use whatever form is most convenient.

Answer 1

The general expression for the quadratic function is :

`f(x)=a(x-h)^2+k`

where, Vertex : (h, k) and h = -b/2a and k = f(h)

In the given question the vertex is ( -1, 3)

Substitute the value of the h = -1 and k = 3

Thus :

`\begin{gathered} f(x)=a(x-h)^2+k \\ f(x)=a(x-(-1))^2+3 \\ f(x)=a(x+1)^2+3 \\ as\text{ the curve passed through : (2, 4) } \\ substitute\text{ x = 2 and at f(2) = 4} \\ f(2)=a(2+1)^2+3 \\ 4=a(3)^2+3 \\ 4=9a+3 \\ 9a=4-3 \\ 9a=1 \\ a=(1)/(9) \\ \text{Substitute the value in the expression :} \\ f(x)=(1)/(9)(x+1)^2+3 \end{gathered}`

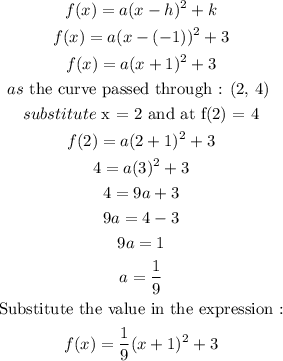
The expression for the quadratic expression is :

f(x) = 1/9(x + 1)² + 3

Answer : f(x) = 1/9(x + 1)² + 3