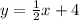step 1
Find out the slope of the given line
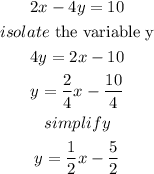
The slope of the given line is m=1/2
step 2
Remember that
If two lines are parallel, then their slopes are equal
that means
The slope of the line parallel to the given line is m=1/2 too
step 3
Find out the equation of the parallel line in slope-intercept form

we have
m=1/2
point (-2,3)
substitute and solve for b
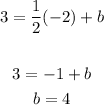
therefore
The equation of the line is