Given the expression:
![\sqrt[]{24(x-1)}/\sqrt[]{8x^2}](https://img.qammunity.org/2023/formulas/mathematics/college/nwhttfjnnrnm08cphf7swh1bi8mhk2mt7d.png)
Let's determine the inequality which represents all values of x where the quotient is defined.
Here, we are to find the domain.
Set the values in the radicand greater or equal to zero and solve for x.
We have:

Set the denominator equal to zero and solve.
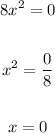
Here, the value of x should not be zero so the denominator will not tend to zero.
Therefore, we have:
x ≥ 1
This means the values of x where the expression is defined must be greater than or equal to 1.
ANSWER:
A. x ≥ 1