Given:
The mass of the block, m=200 g=0.2 kg
The length of the string, L=50 cm=0.5 m
The frequency of rotation of the block, f=75 rpm
To find:
a. Speed of the block.
b. The tension in the string.
Step-by-step explanation:
The angular velocity of the block is,
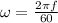
On substituting the known values,
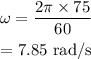
a.
The speed of the block is given by,
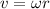
On substituting the known values,
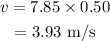
b.
The tension in the string provides the neccessary centripetal force required for the block to trace the circular path.
Thus the tension in the string is given by,
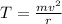
On substituting the known value,
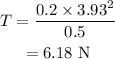
Final answer:
a. The speed of the block is 3.93 m/s
b. The tension in the string 6.18 N