Question

Use the properties of logarithms to write the following expression as a single term that doesn't contain a logarithm.e6-8ln(x)+In(y)

Answer 1

The answer is
`e^(6-8ln(x)+ln(y))`.

Use the properties of logarithms to write the following expression as a single term that doesn't contain a logarithm.

`e^(6-8ln(x)+ln(y))`

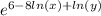
To answer this question, we can use the following properties of logarithms:

`1. e^(ln(a)) = a\\2. e^(a+b) = e^a \cdot e^b\\3. e^(ca) = (e^a)^c`

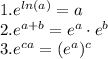
Using these properties, we can rewrite the given expression as follows:

`e^(6-8ln(x)+ln(y)) = e^6 \cdot e^(-8ln(x)) \cdot e^(ln(y))`

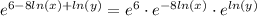
We can then use property 3 to simplify the expression further:

`e^6 \cdot e^(-8ln(x)) \cdot e^(ln(y)) = e^6 \cdot e^(-8ln(x) + ln(y))`

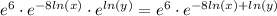
Finally, we can use property 1 to simplify the expression to a single term:

`e^6 \cdot e^(-8ln(x) + ln(y)) = e^(6-8ln(x)+ln(y))`

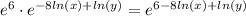
Therefore, the answer to the question is
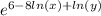`e^(6-8ln(x)+ln(y))`.

Answer 2

Given:

The expression is,

`e^(6-8\ln (x)+\ln (y))`

Step-by-step explanation:

Simplify the expression by using logathimic properties.

`\begin{gathered} e^{6-8\ln (x)+\ln (y)_{}}=e^(6-\ln (x^8)+\ln (y)) \\ =e^6\cdot e^(-\ln (x^8))\cdot e^(\ln (y)) \end{gathered}`

Simplify further.

`\begin{gathered} e^6\cdot e^(-\ln (x^8))\cdot e^(\ln (y))=e^6\cdot(1)/(e^(\ln(x^8)))\cdot e^(\ln (y)) \\ =e^6\cdot(1)/(x^8)\cdot y \\ =(e^6y)/(x^8) \end{gathered}`

So answer is,

`(e^6y)/(x^8)`