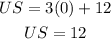Question

In parallelogram STUV, VT bisects US and point G. If UG = x + 6 andGS = 2x + 6, find US.

Answer 1

US = 12

Step-by-step explanation:

The length of GS = UG (because TV bisected the line segment US) and

GS + UG = US

From GS = UG we have

`x+6=2x+6`

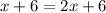
subtracting 6 from both sides gives

`x=2x`

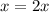
subtracting x from both sides gives

`0=x`

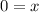
With the value of x in hand, We now find the length of the line segment US.

`US=UG+GS=x+6+2x+6=3x+12`

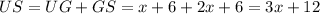
Since x =0, the above gives

`\begin{gathered} US=3(0)+12 \\ US=12 \end{gathered}`