Question

Using the following stem & leaf plot, find the five number summary for the data by hand.1 ---> 482 ---> 3373 ---> 334 ---> 01465 ---> 024476 ----> 13Min =01Med =>Q3-Max =Submit Question

Answer 1

Minimum:

- The minimum is gotten from the value with a stem of 1. The smallest leaf on this stem is 4.

- Thus, the minimum is 14

Q1:

- The first quartile position is gotten using the formula below:

`Q_i=(i(n+1)^(th))/(4)`

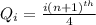
- Thus, the quartile position is:

`\begin{gathered} n=18 \\ Q_1=((18+1))/(4)^(th)=4.75^(th) \end{gathered}`

- Thus, the first quartile is between the 4th and 5th position. This is between 23 and 27.

- To find the correct first quartile, we use the formula below:

`\begin{gathered} 0.75*(27-23)+23 \\ =26 \end{gathered}`

- Thus, Q1 = 26

Median

- The median is gotten below:

`Q_2\text{ or }Median\text{ }position=((n+1))/(2)^(th)=(18+1)^(th))/(2)=9.5^{th\text{ }}position`

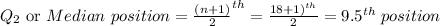
- Thus, the Median is between 41 and 44.

- The median is simply the average of both numbers done below:

`Median=(41+44)/(2)=42.5`

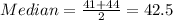
Q3:

- This is the same process as finding Q1. This is done below:

`Q_3\text{ position}=(3(18+1)^(th))/(4)=14.25^(th)`

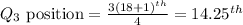
- The Q3 is between 54 and 54.

- Thus, the Q3 is 54 since the Q3 is between the same number

Maximum:

- The maximum value is gotten from the stem with the largest value. The leaf of this stem will be the maximum value of the entire dataset.

- Thus the maximum is 63