Question

Find the equation of the containing given points. Write the equation in slope-intercept form. (7,-9)(9,-9)

Answer 1

The equation of a line passing through two points is expressed as

`\begin{gathered} y-y_1=m\left(x-x_1\right)-----\text{ equation 1} \\ where \\ m\text{ is the slope of the line, which is expressed as} \\ m=(y_2-y_1)/(x_2-x_1)-----\text{ equation 2} \\ where \\ \left(x_1,y_1)\text{ and \lparen x}_2,y_2)\text{ are the coordinantes of the points through which}\right? \\ the\text{ line passes} \end{gathered}`

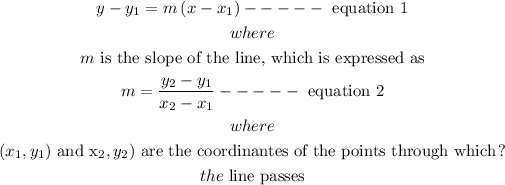
Given that the line passes through the points (7,-9) and (9,-9), this implies that

`\begin{gathered} x_1=7 \\ y_1=-9 \\ x_2=9 \\ y_2=-9 \end{gathered}`

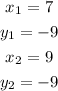
step 1: Evaluate the slope of the line.

Recall that the slope of the line is expressed as

`m=(y_(2)-y_(1))/(x_(2)-x_(1))`

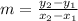
Thus, the slope is evaluated to be

`\begin{gathered} m=(-9-\left(-9\right))/(9-7) \\ =(-9+9)/(9-7)=(0)/(2) \\ \Rightarrow m=0 \end{gathered}`

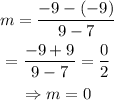
Thus, the slope of the line is zero.

step 2: Express the equation of the line.

Since the slope of the line is evaluated to be zero, we have

In slope intercept form, the equation is expressed as

`\begin{gathered} y=mx+c \\ where \\ m\text{ is the slope} \\ c\text{ is the y-intercept of the line} \end{gathered}`

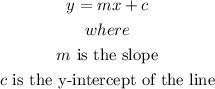
Thus, from equation 3, the equation in slope-intercept form becomes

`\begin{gathered} y+9=0 \\ subtract\text{ 9 from both sides} \\ y+9-9=0-9 \\ \Rightarrow y=-9 \end{gathered}`

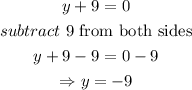
Hence, the equation of the line in slope-intercept form is

`y=-9`