a)
To find the difference quotient we will do
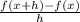
But f(x) = 4x, therefore f(x+h) = 4(x+h). Then
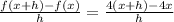
Now we just simplify the expression

Therefore
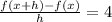
As expected, because the function f is a linear function, then it must be a constant.
b)
As we can see we don't have the term "h" in the difference quotient, then we can easily solve the limit by just repeating the result:
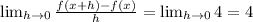
Therefore
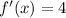
See that it's a constant function, it means that the slope will always be the same, doesn't matter the value of x.