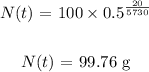Answer:
Step-by-step explanation:
Here, we want to calculate the amount of carbon-14 remaining
The formula to use here is as follows:
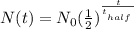
where:
N(t) is the mass left after some time or at a time t which is what we want to calculate
N_0 is the initial mass which is 100g in this case
t is the time which is 20 years
t_half is the half-life which is 5,730 years
Substituting these values, we have it that: