It is given that z varies directly with y and directly with the cube of x.
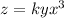
Where k is the constant of proportionality.
First, we need to find the value of k.
It is given that z = 2560 when x = 4 and y = 10

So, the value of k is 4
The relation becomes

Now we can easily find the value of z when x = 8 and y = 4
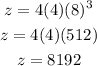
Therefore, the value of z is 8192