Question

Answer part a and part b ill give 5

Answer 1

The given expression is:

`3x^(10)-48x^2`

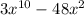
Part a: Factor the two terms as below:

`\begin{gathered} 3x^(10)=3* x* x* x* x* x* x* x* x* x* x \\ 48x^2=3*2*2*2*2* x* x \end{gathered}`

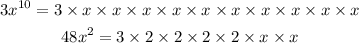
As seen above the common factor is 3 and x and x so it follows:

`\text{GCF}=3x^2`

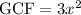
Take the GCF common from the expression to get:

`3x^(10)-48x^2=3x^2(x^8-16)`

Part b: The other bracket can be factored using the formula:

`x^2-a^2=(x-a)(x+a)`

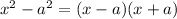
So it follows:

`\begin{gathered} 3x^2(x^8-16)=3x^2((x^4)^2-4^2)=3x^2(x^4-4)(x^4+4) \\ =3x^2(x^4+4)((x^2)^2-2^2) \\ =3x^2(x^4+4)(x^2-2)(x^2+2) \\ =3x^2(x^4+4)(x^2+2)(x-\sqrt[]{2})(x+\sqrt[]{2}) \end{gathered}`

In this way the expression is factored. The final value is the last line given above.