SOLUTION:
Case: Quadrants of trigonometry
Given:
csc θ < 0, tan θ< 0
Required: To find the quadrant the value falls into
Method:
Step 1: First we identify the four(4) major quadrant
Here,
I quadrant. All trigonometry functions are positive
II quadrant. Only sine is positive
III quadrant. Only tangent is positive
IV quadrant. Only Cosine is positive
Step 2:
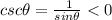
Only the III and IV are negative here.
Step 3: We try the second condition
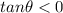
Here only the IV out of the (III) and (IV) will be negative
Final answer:
Only Quadrant (IV) satisfies the condition. Option (D)