Question

To a mass m1 = 100 g of water at θ1 = 10°C we add a mass m2 = 60 g of water at θ2 = 55°C. Calculate the final temperature of the mixture? Data: Specific heat capacity of water in the liquid state: 4.18 kJ.kg -1 .K

Answer 1

Given:

The mass of water is m1 = 100 g = 0.1 kg

The temperature of the water is

`\begin{gathered} \theta1=10^(\circ)\text{ C} \\ =10+273 \\ =283\text{ K} \end{gathered}`

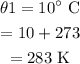
The mass of water is m2 = 60g = 0.06 kg

The temperature of the water is

`\begin{gathered} \theta2=\text{ 55 }^(\circ)\text{C} \\ =55+273 \\ =328\text{ K} \end{gathered}`

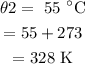
To find the final temperature of the mixture.

Step-by-step explanation:

The final temperature of the mixture can be calculated by the formula

`\begin{gathered} T=((m1\theta1+m2\theta2))/((m1+m2)) \\ \text{ } \end{gathered}`

On substituting the values, the final temperature will be

`\begin{gathered} T=((0.1*283)+(0.06*328))/((0.1+0.06)) \\ =293.625\text{ K} \\ =20.625\text{ }^(\circ)C \end{gathered}`

Thus, the final temperature of the mixture is 20.625 degrees Celsius.