Question

Need help It’s on Plato classesWhich function represents this graph.

Answer 1

D.

EXPLANATION :

From the problem, we have a graph that goes to positive infinity as x goes to the positive infinity.

Note that in an exponential function :

`f(x)=a^x`

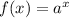
The function goes to positive infinity if "a" is greater than 1

The function goes to negative infinity if "a" is less than 1

Since the given graph goes to positive infinity, we are looking for the function whose "a" is greater than 1.

We have Options A and D.

Next is to check the y-intercept.

The graph intersects the y-axis at (0, -3)

Substitute x = 0 and f(x) should be -3

For Option A :

`\begin{gathered} f(x)=10^x \\ f(0)=10^0=1 \end{gathered}`

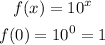
For Option D :

`\begin{gathered} f(x)=10^x-4 \\ f(0)=10^0-4 \\ f(0)=1-4=-3 \end{gathered}`

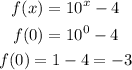
Therefore, the answer is D.