Question

Point W"(3, 5) was created by reflecting W over the line y=x, then rotating it counterclockwise by 90 degrees. What was W?

Answer 1

W(-3,5)

Step-by-step explanation:

Let Point W be the point (x,y)

If W is reflected over the line y=x, we have the transformation:

`(x,y)\rightarrow W^(\prime)(y,x)`

Next, if we rotate W' counterclockwise by 90 degrees, we have the transformation rule:

`W^(\prime)(y,x)\rightarrow W^(\doubleprime)(-x,y)`

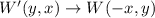
Therefore, we have that:

`\begin{gathered} (-x,y)=(3,5) \\ \implies x=-3\text{ and y=5} \end{gathered}`

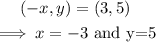
The coordinates of W are (-3,5).