Question

I have a pen filled with sheep and chickens in my back yard. There are a total of 21 animals. If the animals have a total of 56 legs, how many of each type of animal is in the pen?

Answer 1

Let "x" represent the number of sheep and "y" represent the number of chickens.

If there are a total of 21 animals in the pen, we can express the number of animals there with the following expression

`x+y=21`

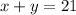
Each sheep has 4 legs, the number of legs per ship multiplied by the number of sheep indicates the total number of sheep legs there are: "4x"

Each chicken has two legs, multiply the number of legs per chicken by the number of chickens to determine the total number of chicken legs: "2y"

With the following expression, we can express the total number of legs in the pen as:

`4x+2y=56`

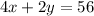
With these two expressions, we have determined a system of equations from which we can calculate the values of x and y.

The first step is to write the first equation for one of the variables, for example, for x:

`\begin{gathered} x+y=21 \\ x+y-y=21-y \\ x=21-y \end{gathered}`

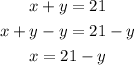
The second step is to replace the expression obtained for x in the second equation this way