Solution:
The probability of an event is expressed as

Given:

In this case, the number of possible outcomes is 20.
Given that the first card is drawn and not replaced before drawing the second card, the probability of selecting a red card followed by a red card is expressed as

For the first red, we have
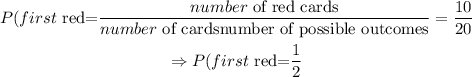
For the second card, we have
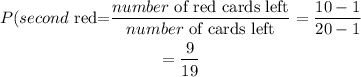
Thus,

Hence, the probability of selecting a red card followed by a red card is evaluated to be
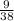
The fourth option is the correct answer.