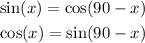The graph of sine and cosine are
The shape are similar. However, there is a 90 shift difference
For instance, when x= 0, we get
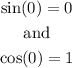
Now, when x=Pi/2 (90 degrees), we get
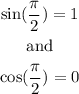
that is, the role has changed when x changes from 0 to 90 degrees. Then, the phase difference between them is 90 degrees (Pi/2)
b) What can you do to the graph of y = cos(x) to obtain the graph of y = sin(x)? We must translate cosine function 90 degrees (Pi/2) to the right, that is
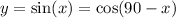
c) Write an equation for the graph of y = sin(x) using a transformation of cosine
From the last relationship,
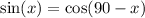
d) Write an equation for the graph of y = cos(x) using a transformation of sine.
In this case, we have the inverse process, that is
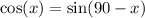
e). Based on your answers to parts (C) and (d), write an equation relating sine and cosine.
The equations are