From the statement of the problem, we have:
• The function A, given by a table of values of x and y,
,
• The function B, given by an equation.
1) For function A, we see that as x increases, the value of y also increases. We also see that the rate of change is constant, given by:

2) For function B, we have the equation:
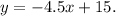
The rate of change of the function is the slope of the line, which is the number that multiplies the variable x. So its rate of change is:

Considering the magnitude of the range of changes, we see that:
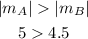
So we conclude that the rate of change of function A is greater than the rate of change of function B.
Answer
Function A has a rate of change of 5 and function B has a rate of change of -4.5, so Function A has a greater rate of change.