Given:
Length of sides of smaller triangle = 4 and x
Lengths of larger sides = 10 and 25
Let's solve for the value of x.
Since both triangles are similar, the corresponding sides will be proportional.
To solve for x, apply the proportionality equation:
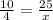
Cross multiply:
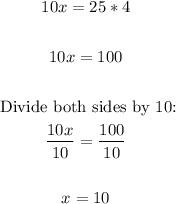
Therefore, the value of x is 10.