Question

The graph of an equation is given. (a) Find the intercepts. (b) Indicate whether the graph is symmetric with respect to the x-axis, the y-axis, or the origin.

Answer 1

The intercepts of a graph are the points where the graph cuts or passes through the axes of the graph.

From the graph given, it has x-intercepts only.

The x-intercept is the point where the graph of the line or curve crosses the x-axis. It has an ordered pair (x, 0), where x is the x-coordinate of the point.

a) The x-intercepts of the graph occurs when y = 0

At y = 0, the x-intercept occurs at;

`\begin{gathered} (-5,0) \\ \\ \text{and} \\ \\ (5,0) \end{gathered}`

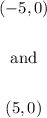
b) A graph is symmetric with respect to the y-axis if, for every point (x,y) on the graph, there is also a point (−x,y) on the graph.

A graph is symmetric with respect to the x-axis if, for every point (x,y) on the graph, there is also a point (x,-y) on the graph.

From the graph given, we have for every point (x,y), a point (-x,y).

Therefore, the graph is symmetric with respect to the y-axis.