Question

A Construction worker needs to pour a rectangular slab 29 feet long 16 feet wide and 6 inches thick how many cubic yards of concrete does the worker need

Answer 1

We have a rectangular slab.

The volume of concrete is equal to the volume of this rectangular prism.

The volume of this prism is equal to the product of its length, width and thickness.

Then, we can write:

`\begin{gathered} V=L\cdot W\cdot t \\ V=29ft\cdot16ft\cdot6in \\ V=29ft\cdot16ft\cdot(6in\cdot(1ft)/(12in)) \\ V=29\cdot16\cdot(6)/(12)ft^3 \\ V=232ft^3 \end{gathered}`

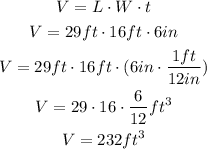
We convert the thickness from inches to feet by multiplying it by a unit ratio 1 ft / 12 inches, which has a value of 1, as 1 ft = 12 inches, but let us change the units from inches to feet.

When we have all the measures in feet we can have the volume in cubic feet.

Answer: 232 cubic feet.