the solution works in both equations
See graph below
Step-by-step explanation:
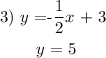
To plot y = 5, it will be an horizontal line on y = 5 that extends towards the negative and positive side of the graph
y = -1/2 x + 3
This is a linear equation. To plot this graph, we will assign values to x in order to get corresponding values to y
let x = -5, 0 5
when x = -5
y = -1/2 (-5) + 3 = 5/2 + 3
y = 5 1/2
when x = 0
y = -1/2 (0) + 3 = 0 + 3
y = 3
when x = 5
y = -1/2 (5) + 3 = -5/2 + 3
y = -1/2
plotting the graph:
The point of intersection is the solution of the graph. The point of intersection is (-4, 5)
x = -4, y = 5
To check if the solution is correct, we will substitute 5 for y in the first equation since the value of y from our solution is 5
y = -1/2 x + 3
5 = -1/2 (-4) + 3
5 = 2 + 3
5 = 5
Since the left and side is equal to right hand side, it is true
We can also test for x
when x = -4
y = -1/2 (-4) + 3
y = 4/2 + 3
y = 2 + 3
y = 5
This is the same value as y in our solution using graph
Hence, the solution works in both equations