The area between the curves is given by:
![A=\int_a^b[|f(x)-g(x)|]dx](https://img.qammunity.org/2023/formulas/mathematics/college/odba25rnqhfuhdgxsxkcz03ro4fpwlf81l.png)
Where a=0 and b=4.
And

Replacing.
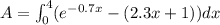
Solving:


Replacing x by the limits:
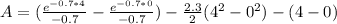
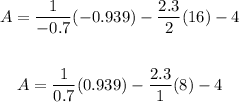
Therefore, the area is:
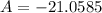
Taking the absolute value:
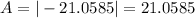
We take absolute value because negative areas make no sense.
Answer: The area between the curves is: 21.06 approximately 21.1.