Question

True or false? linear equations in one variable can be solved algebraically pr graphically

Answer 1

Given: The statement " linear equations in one variable can be solved algebraically pr graphically "

To Determine: If the given statement is true or false

A linear equation is an equation in the form

`\begin{gathered} ax+b=0 \\ \text{Where} \\ a\text{ and b are integers} \\ x\text{ is the variable} \end{gathered}`

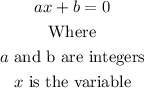
The linear equation has only one solution.

Let us solve algebraically as below

`\begin{gathered} ax+b=0 \\ ax=-b \\ x=-(b)/(a) \\ a=2,b=4 \\ x=-(4)/(2) \\ x=-2 \end{gathered}`

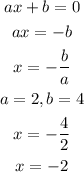
Let us solve graphically

`\text{let a = 2, b = }4`

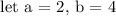
Therefore, the equation becomes

`2x+4=0`

Plot the graph of the equation as shown below

The point where the line cuts the x-axis is the solution of the equation.

Hence

The statement "Linear equations in one variable can be solved algebraically and graphically" is TRUE