Question

For each Arithmetic sequence, identify the next 3 terms of the sequence -2,4,.......

Answer 1

10, 16, 22

Step-by-step explanation:

Pre-Solving

We are given an arithmetic sequence, and we already know that the first two terms are -2 and 4.

We want to find the next three terms in the sequence.

Solving

The formula for determining the nth term of an arithmetic sequence is
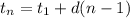`t_n=t_1 + d(n-1)`, where
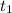`t_1` is the first term and d is known as the common difference.

The common difference can be found by subtracting the second term - first term.

That would be: 4 - -2 = 4 + 2 = 6

So the common difference (d) of this sequence is 6.

We also know that the first term is -2.

Now, we can find the next three terms.

The third term will be:

`t_3 = -2 + 6(3-1)\\t_3 = -2 + 6(2) \\t_3 = -2 + 12\\t_3 = 10`

The fourth term will be:

`t_4 = -2 + 6(4-1) \\t_4 = -2 + 6(3) \\t_4=-2+18\\t_4=16`

The fifth term will be:

`t_5 = -2 + 6(5-1) \\t_5= - 2 + 6(4) \\t_5=-2 + 24 \\t_5 = 22`

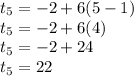
So the next three terms will be: 10, 16, and 22.

Answer 2

10,16 and 22

Step-by-step explanation:

First, we determine what is added to -2 to obtain 4.

`\begin{gathered} -2+x=4 \\ x=4+2 \\ x=6 \end{gathered}`

Therefore, we add 6 to the previous term to obtain the next term.

`\begin{gathered} 4+6=10 \\ 10+6=16 \\ 16+6=22 \end{gathered}`

The next 3 terms of the sequence -2,4,... are 10,16 and 22.