Question

Select the correct answer. What is the solution to this system of equations? 3a + 40 + 2c = 11 2a + 3b – c = 4 - C 50 + 50 – 3c = -1 - A. a = 2, b = 3, c = 4 3 a = 4, b = 2, c = 3 2 B. 4,8 OC. G = -3, b = 4, c = 2 4 D. a = -4, -4, b = -3, c = -2

Answer 1

The equations are given below as

`\begin{gathered} 3a+4b+2c=11\ldots\ldots(1) \\ 2a+3b-c=4\ldots\text{.}(2) \\ 5a+5b-3c=-1\ldots..(3) \end{gathered}`

Step 1:

Multiply equation 1 by 2 and multiply equation 2 by 3

`\begin{gathered} 2(3a+4b+2c)=11*2 \\ 6a+8b+4c=22\ldots\text{.}(4) \\ 3(2a+3b-c))=4*3 \\ 6a+9b-3c=12\ldots\text{.}(5) \end{gathered}`

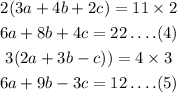
Step 2:

Substract equation 4 from 5

`\begin{gathered} 6a-6a+9b-8b-3c-4c=12-22 \\ b-7c=-10\ldots\text{.}(6) \end{gathered}`

Step 3:

Multiply equation (2) by 5 and then equation 3 by 3

`\begin{gathered} 5(2a+3b-c)=4*5 \\ 10a+15b-5c=20\ldots\ldots(7) \\ 2(5a+5b-3c)=-1*2 \\ 10a+10b-6c=-2\ldots\text{.}(8) \end{gathered}`

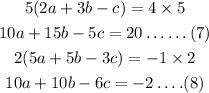
Step 4:

Substract equation (8) from equation (7)

`\begin{gathered} 10a-10a+15b-10b-5c+6c=20+2 \\ 5b+c=22\ldots\ldots(9) \end{gathered}`

Step 5:

From equation (9)

`c=22-5b\ldots\text{.}(10)`

Substitute equation 10 in equation (6)

`\begin{gathered} b-7c=-10 \\ b-7(22-5b)=-10 \\ b-154+35b=-10 \\ 36b=-10+154 \\ 36b=144 \\ (36b)/(36)=(144)/(36) \\ b=4 \end{gathered}`

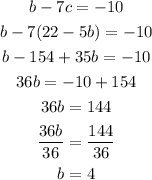
Step 6:

Substitute the value of b=4 in equation (10)

`\begin{gathered} c=22-5b \\ c=22-5(4) \\ c=22-20 \\ c=2 \end{gathered}`

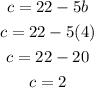
Step 7:

Substitute c=2 and b=4 in equation (2)

`\begin{gathered} 2a+3b-c=4 \\ 2a+3(4)-2=4 \\ 2a+12-2=4 \\ 2a+10=4 \\ 2a=4-10 \\ 2a=-6 \\ (2a)/(2)=-(6)/(2) \\ a=-3 \end{gathered}`

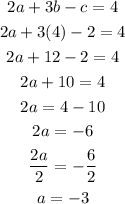
Hence,

a =-3 , b=4 , c=2

OPTION C is the right answer