Question

Find the distance between the foci of an ellipse. The lengths of the major and minor axes are listen respectively. 50 and 14

Answer 1

48

EXPLANATION :

The length of the major axis (2a) is 50 and the length of the minor axis (2b) is 14

So :

`\begin{gathered} 2a=50 \\ a=25 \\ \\ 2b=14 \\ b=7 \end{gathered}`

The distance between the foci is 2c, and the formula of c is :

`c^2=a^2-b^2`

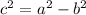
Using the formula above :

`\begin{gathered} c^2=25^2-7^2 \\ c^2=576 \\ c=√(576) \\ c=24 \end{gathered}`

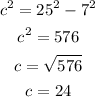
Then the distance of the foci is 2c = 2(24) = 48