In order to find the correct graph, first let's find some points of the function f(x):
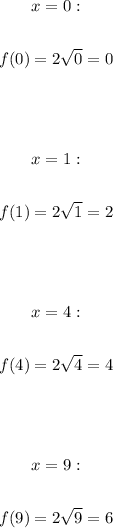
So the function f(x) has the points (0, 0), (1, 2), (4, 4) and (9, 6).
The inverse function must have the same points with the coordinates changed, that is, the points (0, 0), (2, 1), (4, 4) and (6, 9).
Therefore the correct option is the third one.