Let the given values represent t and f(t), where t is the x-coordinate and f(t) is the y-coordinate. Thus, the given values are points on the function:
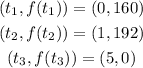
Note that f(t) represents the height of ball after t seconds.
Suubstitute the values of t and f(t) for x and y, respectively, in the quadratic equation:

Thus, we have the following system of equations:
Substituting (0,160) into the equation, we have:
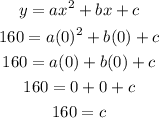
Substituting (1,192) and c = 160 into the equation, we have:
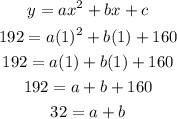
Substituting, (5,0) and c = 160 into the equation, we have:
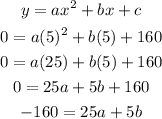
Multiply each term on both sides of the the equation 32 = a + b by -5.
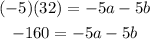
Add the obtained equation to -160=25a+5b to eliminate the variable term 5b and then divide both sides by 20 to find a.

Substitute the value of a into 32 = a+b and then add both sides of the equation by 16 to find the value of b.
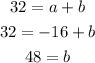
Substitute the obtained values of a, b, and c into the quadratic equation.

To recheck, graph the equation and determine if all of the given points are on the graph. The graph of the equation is as follows:
Note that the graph of the equation in the problem is represented on the Quadrant I only.
Since all of the points are on the equation, the quadratic equation which represents the path of the ball must be y = - 16x² + 48x + 160, where x is a non-negative number.