4. Reflect across the line y = x ------- (b,a)
for reflection across the line y=x, the value of x and y coordinates are interchanged;
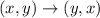
5. Reflect across any horizontal line. Ex: y = -2 ......... (a,2y-b)
for reflection across any horizontal line, the x coordinate remain the same but the y coordinate is subtracted from twice the value of the y coordinate of the line of reflection.
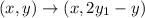
6. Reflect across any vertical line. Ex: x = 5 ---------- (2x-a, b)
for reflection across any vertical line, the y coordinate remain the same but the x coordinate is subtracted from twice the value of the x coordinate of the line of reflection.
