Question

The value of a in y = ax²+bx+c and the vertex of the parabola are given. How many x-intercepts does the parabola have? Explain how you arrived at this number.a=1; vertex at (2,0)The parabola has x-intercept(s), because the parabola opensand the vertex isthe x-axis.

Answer 1

Given: The value of 'a' in y = ax²+bx+c is a=2 and vertex is at (2,0).

Required: To find the x-intercepts.

Explanation: The x-coordinate of the vertex is 2. Also, we know that x-coordinate is given by

`x=-(b)/(2a)`

Hence, putting the value of x=2 and a=1 we get

`\begin{gathered} 2=-(b)/(2(1)) \\ b=-4 \end{gathered}`

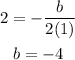
Now putting y=0, x=2, a=1, and b=-4 in eq of parabola we get

`\begin{gathered} 0=2^2-4(2)+c \\ c=4 \end{gathered}`

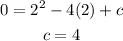
Now the equation of the parabola is,

`y=x^2-4x+4`

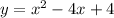
Now to find x-intercepts put y=0 i.e.,

`\begin{gathered} x^2-4x+4=0 \\ (x-2)^2=0 \\ x=2,2 \end{gathered}`

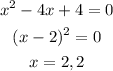
Hence there is only one x-intercept at (2,0). The opening of the parabola can be seen in the graph below-

Final Answer: The parabola has one x-intercept because the parabola opens upward and the vertex is on the x-axis.