The floor of a moving van is 3 ft off the ground.
The ramp into the van makes a 12-degree angle with the ground.
How long is the ramp?
Let us draw a figure to better understand the problem.
As you can see from the above figure,
In the above triangle, with respect to angle 12°, the opposite side is 3 ft and the hypotenuse is x
The hypotenuse (x) is the length of the ramp that we need to find.
Recall from the trigonometric ratios,
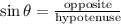
For the given case,
θ = 12°
Opposite = 3
Hypotenuse = x
Let us substitute these values into the above formula

Therefore, the ramp is 14.43 ft long.