Question

ofThis diagram shows a pre-image A ABC, and its image,AA"B"C", after a series of transformations,Select from the drop-down menus to correctly complete thestatements7+6+5+4+A ABC is Choose...to become3+Bс4:27A A'B'C'. Then AA'B'C' is1 +HA-8-7 to 5ChoosetoB-3 -2 -1 3 À 6 i åA2ABbecome A A"B"C" Because the transformations areС-3+-4+Choose...the pre-image and image are--5+C-6+VChoose-7+-8+

Answer 1

The following were the observed points of the triangles as shown in the image provided:

`A(1,-1),B(4,-2),C(7,2)`
`\begin{gathered} A^(\prime)(-1,1) \\ B^(\prime)(-4,2) \\ C^(\prime)(-7,-2) \end{gathered}`
`A^(\doubleprime)(-1,-2);B^(\doubleprime)(-4,-1);C(-7,\text{ -5)}`

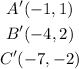
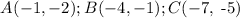
The transformation of triangle ABC to A'B'C' is of the rule (x, y) to (-x, -y). This rule represents a 180-degree counterclockwise rotation

The transformation of triangle A'B'C' to A"B"C" is of the rule (x, y) to (x, y-3). This rule represents a translation down by 3 units

Hence, ABC is rotated 180 degrees counterclockwise about the origin to become A'B'C'. Then A'B'C' is translated 3 units down to become A"B"C" the transformation are both rigid, the pre-image and image are congruent