Question

Find the angle between the vectors, approximate your answer to the nearest tenth: v*(-4,-3) , w = (2,6)w145.3°O 108.4034.7°O 71.6°

Answer 1

`\theta=\text{ 145.3\degree}`

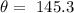
Explanation:

The angle between two vectors is represented by the following equation:

`\cos \theta=\frac{\vec{u}\cdot\vec{v}}{\lvert\vec{u}\rvert\lvert\vec{v}\rvert}`

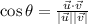
Notice that it involves a trigonometric function, the dot product of two vectors, and the magnitude of two vectors.

Then, let's determine the dot product of the two vectors:

`\begin{gathered} \vec{v}\cdot\vec{w}=-4\cdot2+-3\cdot6 \\ \vec{v}\cdot\vec{w}=-26 \end{gathered}`

Now, calculate the magnitudes of the vectors:

`\begin{gathered} \lvert\vec{v}\rvert=\sqrt[]{(-4)^2+(-3)^2}=5 \\ \lvert\vec{w}\rvert=\sqrt[]{(2)^2+(6)^2}=2\sqrt[]{10} \end{gathered}`

Now, substitute into the equation to find the angle:

`\begin{gathered} \cos \theta=\frac{-26}{5\cdot2\sqrt[]{10}} \\ \theta=\cos ^(-1)(\frac{-26}{5\cdot2\sqrt[]{10}}) \\ \theta=\text{ 145.3\degree} \end{gathered}`