Answer:
B
Explanation:
The range of a function f, would contain outputs that the function can output, or in other words, y-values.
Generally we use inequalities to describe functions that are continuous (not always the case) over a certain interval because using inequalities, doesn't just mean that the inequality has some outputs, but rather every value that satisfies the given inequality, should be an output.
For example if the function f(x) only outputs: 1, 2, 3, 4
It would be invalid to say the range is:
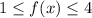
because saying this is the range, would imply that all values between 1 and 4 (including 1 and 4), are in the range. Meaning that 1.2, 1.3, 1.4, etc... are also in this range, but they're not since the function doesn't output these y-values.
Now looking onto the graph provided, we only want to focus on the outputs aka y-values.
Looking at the graph, we can see that the y-values are: 1, -4, 5, -3, and -3.
Notice that we actually have a duplicate y-value, and whenever we have this, we don't state it "twice" in the range, since the range is just telling us of y-values the function can output, and not how many times it occurs.
Thus the range is: -4, -3, 1, and 5
So "b" would be the correct option.
Note: "D" would be the correct choice for the domain of this function (like the range, except it deals with x-values)