We have
The formula of the volume of a rectangular prism
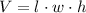
the formula of the volume of a pyramid
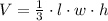
The formula of the volume of a cylinder is
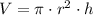
The formula of the volume of a cone is
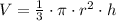
As we can see following the formulas above
C. The volume of a pyramid is one-third the volume of a rectangular solid with the same base and height, just as the volume of a cone is one-third the volume of a
cylinder with the same base and height