Question

Help 4 tutors skipped because they didn’t think it was math… IT IS MATH

Answer 1

Let the chemist mix 'x' litres of the first solution and 'y' litres of the second solution.

Given that the resulting solution is 60 litres,

`x+y=60\ldots(1)`

Consider that the first solution is 45% concentrated, so the amount of salt in 'x' litres of the first solution will be 45% of 'x',

`\begin{gathered} =(45)/(100)\cdot x \\ =0.45x \end{gathered}`

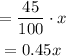
Similarly, the second solution is 30% concentrated, so the amount of salt in 'y' litres of the second solution will be 30% of 'y',

`\begin{gathered} =(30)/(100)\cdot y \\ =0.30y \end{gathered}`

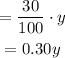
The resulting solution is 40% concentrated, so the amount of salt in 60 litres of the resulting solution will be 40% of 60,

`\begin{gathered} =(40)/(100)\cdot60 \\ =24 \end{gathered}`

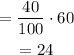
Since the amount of salt must remain the same before and after the mixing, the sum of amounts of salt from the first and second solution should be equal to 24,

`\begin{gathered} 0.45x+0.30y=24 \\ 3x+2y=160\ldots(2) \end{gathered}`

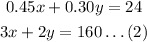
Multiplying equation (1) by 2 and subtracting from equation (2),

`\begin{gathered} (3x+2y)-2(x+y)=160-2(60) \\ 3x+2y-2x-2y=160-120 \\ x+0=40 \\ x=40 \end{gathered}`

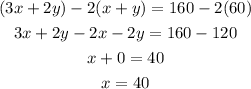
Substitute this value in equation (1),

`\begin{gathered} 40+y=60 \\ y=20 \end{gathered}`

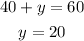
Thus, it can be concluded that the chemist mixed 40 litres of the 45% solution and 20 litres of the 30% solution.

Therefore, 4th option is the correct choice.