You have the following function for the height of debris:
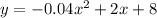
In order to answer the given questions, consider that the graph of the given function is:
1) The distance at which the debris land is given by one of the zeros of the function, because y is a parabolla, as you can notice in the previous image.
Use the quadratic formula to find the zeros of the function:
![x=\frac{-b\pm\sqrt[]{b^2-4ac}}{2a}](https://img.qammunity.org/2023/formulas/mathematics/college/rxvf73usjbbwyik14knxdemoz21vfz2ufc.png)
coefficients a, b and c are the coefficients of the quadratic function ax^2+bx+c. In this case, by comparing with the given expression for y, you have:
a = -0.04
b = 2
c = 8
Replace the previous values into the quadratic formula and simplify:
![\begin{gathered} x=\frac{-2\pm\sqrt[]{(2)^2-4(-0.04)(8)}}{2(-0.04)} \\ x=\frac{-2\pm\sqrt[]{4+1.28}}{-0.08} \\ x=\frac{-2\pm\sqrt[]{5.28}}{-0.08} \\ x=(-2\pm2.297825059)/(-0.08) \end{gathered}](https://img.qammunity.org/2023/formulas/physics/college/klhia8vyh6k70mvfatxgkw8o6ze8f352jv.png)
select only the positive result because it has physical meaning:

Hence, the debris are at a distance of 53.72281323 feet from the launching site.
2) The maximum height reached by fireworks, is represented by the y-coordinate of the vertex point of the parabolla (as you can notice in the graph).
The x-coordinate of the vertex of a parabolla is given by:

Replace the values of a and b, and simplify:
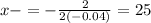
The value of y for the previous value of x is:
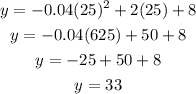
The previous value is the y-coordinate of the vertex.
Hence, the maximum height reached by the fireworks is 33 feet