The solution of the equation expressing it as a logarithm in base-e is t = ln (6.714)/0.1.
The approximate value of t = 19.042.
How to calculate the value of t?
The solution of the equation expressing it as a logarithm in base-e is calculated as follows;
The given equation;
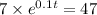
We will divide both sides of the equation by 7;

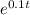 = 6.714
= 6.714
Take log of both sides of the equation;
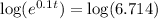
0.1t (ln e) = ln (6.714)
t = ln (6.714)/0.1
The approximate value of t is calculated as;
t = ln (6.714)/0.1
t =1.9042 / 0.1
t = 19.042
t ≈ 19.042