Question

At the book store, you purchased some $3 clearance mystery books and $8regular-priced science fiction books. How many of each did you buy if you spent atotal of $77?

Answer 1

In order to find how many of each books we bought, let's use the variable x to represent the number of clearance mystery books and the variable y to represent the number of science fiction books.

Using these variables and the cost of each book, we can write the following equation:

`3x+8y=77`

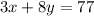
Since we have an equation with two variables, we can have multiple solutions for the equation.

The natural solutions are:

`\begin{gathered} x=7,y=7\colon \\ 3\cdot7+8\cdot7=77 \\ 21+56=77 \\ 77=77 \\ \\ x=15,y=4\colon \\ 3\cdot15+8\cdot4=77 \\ 45+32=77 \\ 77=77 \\ \\ x=23,y=1\colon \\ 3\cdot23+8\cdot1=77 \\ 69+8=77 \\ 77=77 \end{gathered}`

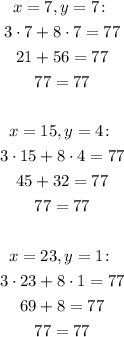
So with $77 we can buy:

- 7 clearance mystery books and 7 science fiction books,

- 15 clearance mystery books and 4 science fiction books,

- 23 clearance mystery books and 1 science fiction book,