Question

Suppose that you and a friend are playing cards and decide to make a bet. If you draw three black cards in succession from a standard deck of 52 cards without replacement, you win $50. Otherwise, you pay your friend $20. What is the expected value of your bet? Round your answer to the nearest cent, if necessary.

Answer 1

-11.76 dollars

Explanation

First, we need to calculate the probability of winning, that is, the probability of drawing three black cards in succession without replacement.

In the beginning, there are a total of 52 cards, and 26 of them are black, then the probability that the first card drawn is black is:

`P(first\text{ card is black})=(26)/(52)=(1)/(2)`

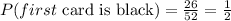
After drawing 1 black card, there are a total of 51 cards, and 25 of them are black, then the probability that the second card drawn is black is:

`P(\text{ second card is black})=(25)/(51)`

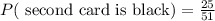
After drawing 2 black cards, there are a total of 50 cards, and 24 of them are black, then the probability that the third card drawn is black is:

`P(\text{ third card is black})=(24)/(50)=(12)/(25)`

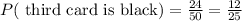
Therefore, the probability of winning, that is, the three cards are black is calculated as follows:

The probability of losing is the complement of the probability of winning, that is,

`\begin{gathered} P(\text{ losing})=1-P(winning) \\ P(\text{los}\imaginaryI\text{ng})=1-(6)/(51) \\ P(\text{los}\mathrm{i}\text{ng})=(45)/(51) \end{gathered}`

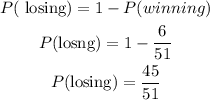
Finally, the expected value is calculated as follows:

`\begin{gathered} EV=P(winning)\cdot\text{ Amount won per bet}-P(losing)\cdot\text{ Amount lost per bet} \\ EV=(6)/(51){}\cdot\text{ \$}50\text{ - }(45)/(51)\cdot\text{ \$}20 \\ EV=-\text{ \$}11.76 \end{gathered}`