Question

Identify the intercepts of the function f(x) = x2 - 2x - 15.

Answer 1

The x intercepts are ( -5,0) and (3,0)

The y intercept is ( 0,-15)

Step-by-step explanation:

f(x) = x^2 - 2x - 15.

To find the x intercepts, let the function equal zero

0 = x^2 - 2x - 15

Factor

0 = ( x-5) (x+3)

Using the zero product property

x-5 =0 x+3 =0

x=-5 x=-3

The x intercepts are ( -5,0) and (3,0)

To find the y intercept, let x=0

y = 0^2 -2(0) -15

y = -15

The y intercept is ( 0,-15)

Answer 2

C) the x-intercepts: (-3, 0) and (5, 0); y-intercept (0, -15)

Step-by-step explanation:

The y-intercept of the function is the value of f(x) when x is equal to 0, so if we replace x by 0, we get:

f(x) = x² - 2x - 15

f(0) = 0² - 2(0) - 15

f(0) = 0 - 0 - 15

f(0) = -15

Therefore, the y-intercept is the point (0, -15)

On the other hand, the x-intercepts are the values of x when f(x) is equal to zero. So, to find the x-intercepts, we need to solve the following equation:

f(x) = x² - 2x - 15 = 0

x² - 2x - 15 = 0

So, if we factorize the expression we get:

`\begin{gathered} x^2-2x-15=0 \\ (x-5)(x+3)=0 \end{gathered}`

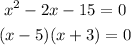
Then, there are two possible options:

x - 5 = 0

x - 5 + 5 = 0 + 5

x = 5

Or

x + 3 = 0

x + 3 - 3 = 0 - 3

x = - 3

Therefore, the x-intercepts of the equation are the points (-3, 0) and (5, 0)

So, the answer is C) the x-intercepts: (-3, 0) and (5, 0); y-intercept (0, -15)